
This REU program was funded through NSF PHY-1263201.
Students' names link to their final papers. Advisors' names link to the research group web pages.
Condensed Matter, Experiment and Theory
Quantized vortices in superfluid helium play a key role in superfluid turbulence. At the lowest temperatures, the mechanism for transfering energy from larger to smaller length scales may rely on nonlinearities in oscillations along the vortices. Ryan Gnabasik (Creighton University; advisor Rena Zieve) set up continuous wave excitation measurements for a vibrating wire surrounded by superfluid. Previously the lab had excited vibrations with a sharp pulse and monitored their ensuing decay. When a superfluid vortex is partially attached to the wire, its position can be determined from a beat frequency observed during the decay. Ryan instead measured the wire's response to a fixed-amplitude excitation as a function of frequency. This gives the Fourier transform of the pulsed measurement and contains the same information. After mapping out the frequency behavior, Ryan showed that monitoring at a carefully chosen frequency can track vortex dynamics on a faster timescale than the previous technique. The method provides an opportunity to study directly the oscillatory normal modes of a single superfluid vortex, including nonlinear effects.
Alex Dorsett (Butte Community College; advisor Shirley Chiang) used low-energy electron microscopy to monitor the behavior of gold atoms on a germanium surface. The total amount of gold was enough to cover about half the surface in a single layer. In practice though, the gold clumped into islands. Upon increasing the temperature, the islands both coalesced into larger islands and also elongated along a particular crystal direction of the underlying germanium. Reducing the temperature eliminates the elongation, but the islands remain large, showing that the large size is energetically favorably but difficult to reach directly at the lower temperatures. Future work will investigate how to control growth of particular structures.
Experiments probing granular behavior must be highly automated, since effects are often statistical and require analysis of large numbers of images. Brendan Lockhart (University of Maryland; advisor Rena Zieve) worked on debugging analysis software that had been ported from IDL to Python. The software identifies the locations and colors of ball bearings within a two-dimensional pile, then uses this information to resolve which bearings have been welded together into hexagonal clusters. Brendan suggested and wrote a new algorithm for locating the ball centers.
Topological insulators (TIs) are three-dimensional samples that are insulating in the bulk but have special surface states. That materials can have very different properties at their surface is not so surprising; after all, the surface atoms are missing many of the neighboring atoms that would be present away from the sample's edge, which changes their electronic and possibly magnetic environment. The great interest in TIs comes because there is surface conduction which is both spin-polarized (spin-up and spin-down electrons travel in different regions of the surface) and also highly resistant to the scattering that typically degrades currents. Hence TIs may be ideal conduits in developing spintronics, an analog of electronics based on spin rather than charge. Andrea Gaughan (Haverford College; advisor Nick Curro) grew samples of a particular TI, bismuth selenide, and performed nuclear magnetic resonance (NMR) measurements on them. The NMR work probed the energies and lifetimes of spin states in the bulk of the material. Any ultimate applications will require detailed knowledge of bulk properties and of the interactions between the bulk and surface states.
Vanadium dioxide is insulating at room temperature but undergoes a
first-order transition to a metallic state at the moderately
elevated temperature of 68°C. The resistance change of several
orders of magnitude leads to applications as a switch or sensor.
A new method of synthesizing vanadium dioxide produces wires so
thin that they behave in some respects as one-dimensional. Luke Hellwig (Carlton College; advisor Dong Yu) successfully
repeated this growth technique to make vanadium dioxide nanowires, as
shown below, scattered among much larger contact pads and wires. Luke
then characterized the wires with electrical and optical techniques. His
wires were a factor of 10 thinner than those previously reported, and
for many of them holes rather than electrons dominated the conductance.
The metal-insulator transition was also higher than for the bulk material.
These differences may come from slight changes in the growth method.
Varying the growth parameters may allow control over some of these
properties and perhaps new applications.
Trithep Devakul (Northeastern University; advisor Rajiv Singh) looked at possible ground states for fermions that partially fill the sites of a lattice. He began with a Hamiltonian giving the energy of the system in terms of several effects: "hopping" between neighboring sites, favoring of particular sites in the lattice over others, and repulsion between fermions on neighboring sites. He then calculated the favored arrangement of the fermions on the lattice. Depending on the relative strength of the different energy terms, he found different types of behavior corresponding to Fermi liquids or charge density waves. These calculations entirely ignore the spin degree of freedom, which can be reasonable even for fermions if all the particles happen to have identical, constant spin. (Trithep's write-up is in an unusual form, with a single page of introduction preceding an actual published paper. Expect it to be less readable for undergraduates than most of the other papers!)
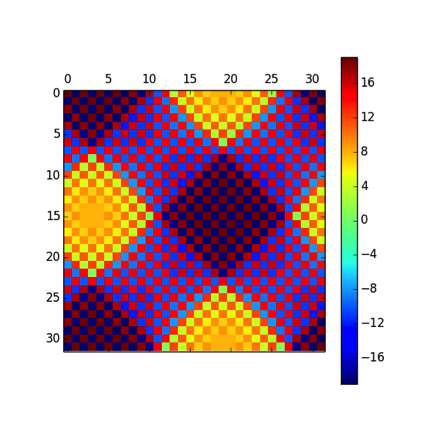
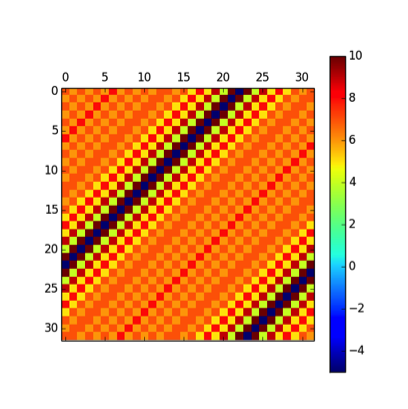
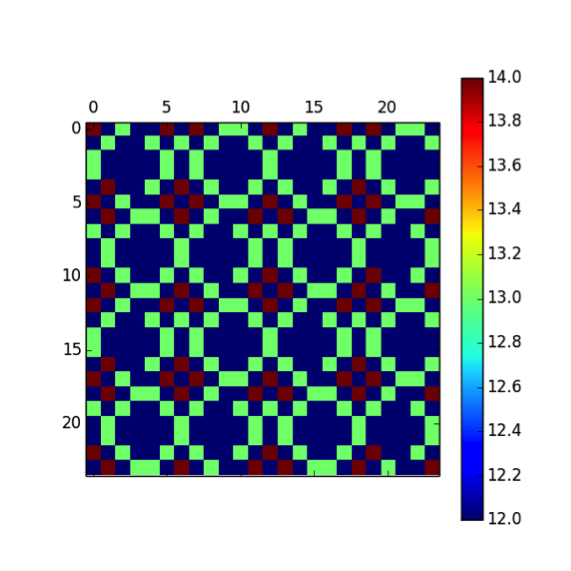
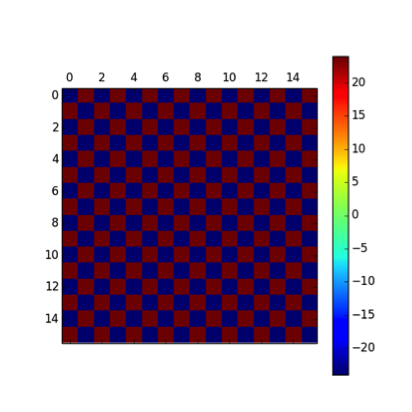
Denisa Goia (University of Wisconsin, Whitewater; advisor Warren Pickett) looked into the problem of using systems with a few hundred or a few thousand atoms to model real-world systems with Avogadro's number of atoms. She looked at how the lowest-energy configurations of electrons on a two-dimensional lattice changed when the size of the lattice and the number of electrons were scaled up together. The ground state often had a clear pattern: rings, stripes, checkerboard, etc. Several of these appear in the images at the top of this page. The lattice size indeed has a significant effect on the pattern, as in one case where clear order for small lattices disappears for larger lattices. Incommensurate polarization levels, where the difference between up-spin and down-spin electrons is not a factor of the lattice size, seem to be influenced less by the change in lattice size, although even in this case there can be some effect.
Thomas Gunn (University of West Florida; advisor Warren Pickett) did computer simulations to model a Kondo lattice, or a lattice of atomic spins within a crystal. In a metal, the response to a single atomic spin is understood. At low temperatures there is spin-dependent scattering of the metal's conduction electrons, which reduces conductivity. However, the conduction electrons can act collectively to screen the influence of the spin. How the electrons behave when faced instead with a regular aray of spins is not understood, but it appears to be a complicated problem that can lead to various magnetic behaviors and even superconductivity. Thomas used an energy function that consider electron kinetic energy (freedom to move between neighboring lattice sites) and Coulomb repulsion (energy cost of having two electrons on the same site). Using a mean-field approximation that used averaged rather than exact electron configurations in some parts of the calculation, he then found minimum-energy electron configurations, which did have a tendency towards magnetic order.
Complex Systems
Alli Nilles (Colorado School of Mines; advisor Jim Crutchfield) studied learning, particularly how a robot can learn about an unfamiliar environment that it explores. She analyzed data from a robot that could move straight ahead or make right-angle turns in either direction. The robot moved on a white piece of paper, with "walls" indicated by thick black lines that the robot would detect and not cross. One reason to use an actual robot rather than simulated data is that reality tends to introduce non-random errors; for example, this robot tended to arc slightly to the right when nominally moving straight ahead. The robot "learned" the odds that its different actions would result in having a wall nearby, and on which side. Alli compared how quickly the robot learned when its actions were selected randomly or through a particular non-random algorithm. In principle a well-chosen algorithm should be better, but in practice random is hard to beat and in this case the two algorithms did comparably well. Alli also constructed simplified graphs of the robot's behavior in rectangular, triangular, and circular rooms, which could help humans to identify actual shapes from the robot's information.
Nuclear and Particle Physics Experiment
One possible form for dark matter is weakly interacting massive
particles (WIMPs). Attempts to observe WIMPs involve large containers
of a heavy liquid. If a WIMP scatters off one of the nuclei within
the liquid, it can produce photons and electrons which are then
detected by photomultiplier tubes and scintillators around the
outside of the chamber. An important question is the lifetime of
an electron within the liquid; if it is too short, then electrons
from events that occur far from the appropriate detectors may be
missed, and those events may be misidentified.
Fransheska
Berrios (California Lutheran University; advisor Mani Tripathi )
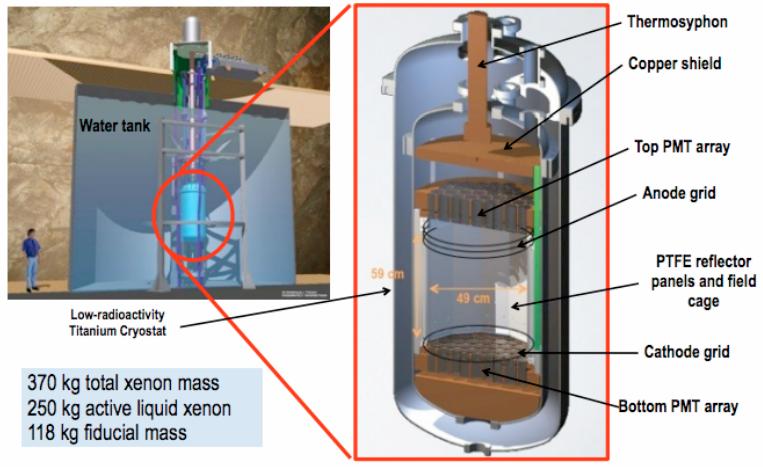
analyzed data from the Large Underground Xenon (LUX) setup. A schematic
of LUX appears on the left below; the human at the far left shows the
scale. Fransheska
found that usually the electron lifetime was about 800 microseconds, well
over the 340 microseconds corresponding to the longest path an electron
would have to traverse. However, two runs showed a much shorter lifetime
near 300 microseconds. The key difference appears to be circulation of
the fluid, which helps to filter out the impurities that can scatter
electrons. The circulation was not functioning properly during two runs
with shortened time constant.

Neutrino experiments and dark matter searches must be able to eliminate background events, from cosmic rays and other undesired radiation. This requires carefully characterizing the detectors, so that unwanted signals can be identified and discarded. Kristyn Brandenburg (Clemson University; advisor Emilija Pantic) used the campus cyclotron to measure the response of a liquid organic scintillator to gamma rays and neutrons. She then analyzed how best to distinguish between these types of radiation, based on the shape of the pulse observed in the scintillator. Typically the "long-time" tail of the pulse (where long means a few hundred nanoseconds!) is a larger fraction of the total integrated pulse for neutrons than for gamma rays. In fact, for sufficiently low cyclotron energies the fraction of the total weight in the pulse tail can accurately identify gammas and neutrons. The right image above plots events by their total amplitude and the contribution of the tail; the neutrons compose the upper strip of points and the gammas the lower strip. For higher energies increased variation in pulse shape prevents identifying the type of radiation with as much certainty.
Colliding heavy ions, such as the gold ions used in Brookhaven National Lab's Relativistic Heavy Ion Collider (RHIC), can briefly free quarks from nuclei into a quark-gluon plasma. As scientists study the resulting state, one important question is determining the exact conditions for the transition from bound to free quarks. This requires lowering the energy in collisions at RHIC below its present level. One way to do so is to consider collisions of a single ion with a fixed target, rather than head-on collisions between two ions. A current setup at RHIC aims to monitor both types of events simultaneously, in different parts of the beam. Erin Hodson (University of Texas, El Paso; advisors Manuel Calderon de la Barca Sanchez and Daniel Cebra) analyzed early data to test whether the outside of the beam, which collides with the fixed target, contains gold ions. She found that this portion of the beam definitely contains heavy ions, rather than lighter ions from the imperfect vacuum in the beam tube. A final step will be to use collision data to show that these heavy ions are indeed gold. If so, the fixed gold will enable comparisons of gold-gold collisions at widely different energies, and may pinpoint the transition to the quark-gluon plasma.
Jayss Marshall (American River College; advisor Mani Tripathi ) worked to identify Compton scattering events in a germanium gamma-ray detector. Compton scattering is misleading in that not all of the photon's energy is deposited in the germanium. In another type of interaction, the photoelectric effect, all the energy is transfered to an electron. The third electron-photon interaction, the annihilation of a photon in creation of an electron-positron pair, occurs at too high energies to matter in this experiment. By adding an additional scintillator and photomultiplier tubes, Jayss was able to detect photons leaving the germanium detector after a Compton scattering event. He could then mark the events in the germanium detector corresponding to incomplete energy transfer, and by discarding these events could obtain a better energy distribution for the original gamma rays.
The quark-gluon plasma is observed both at the Relativistic Heavy Ion Collider in Brookhaven and at the Large Hadron Collider in Geneva. Comparing results between the two machines is important both for confirmation of the basic physical picture and because they probe different energy regimes. Brandon McKinzie (American River College; advisors Manuel Calderon de la Barca Sanchez and Daniel Cebra) analyzed upsilon mesons resulting from heavy-ion collisions. He found that the dependence of upsilon yield on the number of charged particles at RHIC at least resembles that observed at the LHC. The quality of the RHIC data does not yet allow a good quantitative comparison.
Astrophysics
A theme through many fields of physics is that as more powerful machines (telescopes, accelerators, computers, etc.) increase the rate of data acquisition, analysis must be increasingly automated. One place this appears in astronomy is in identifying objects as stars in the Milky Way or as very distant galaxies. For any individual object, detailed measurements can make the distinction obvious. However, carrying out such measurements for every object detected by survey telescopes is impractical. Instead, Victoria Strait (Furman University; advisor Tony Tyson) developed an algorithm that usually identifies an object correctly as star or galaxy. The algorithm examines the brightness of an object in different color ranges. Victoria looked for color pairs that separate stars and galaxies the most cleanly. Her algorithm identifies each object and also assigns a probability that the identification is correct. This information can then be used when astronomers turn to the survey data to select groups of stars or of galaxies for further study.